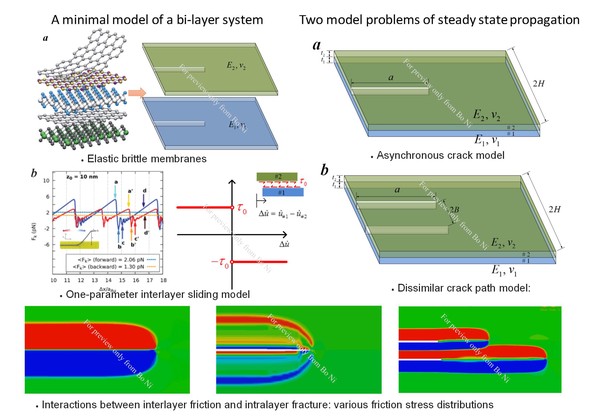
Fracture under interlayer sliding in multilayered 2D materials
2D materials communicate with the environment through large area of surface. Recent experimental measurements have deepened our understanding of the friction between layers of 2D materials and suggested the interlayer properties, such as interlayer friction, can couple with intralayer properties and affect the overall behavior of multilayered 2D material systems. In this study, the effect of interlayer friction on asynchronous crack propogation and dissimilar crack paths is considered by integrating theoretical analysis and numerical simulation. It is found that the intact layer can postpone crack propogation in the neighbouring layer and cracks along dissimilar paths can communicate via interlayer sliding zone, resulting in a size-dependent fracture behavior.
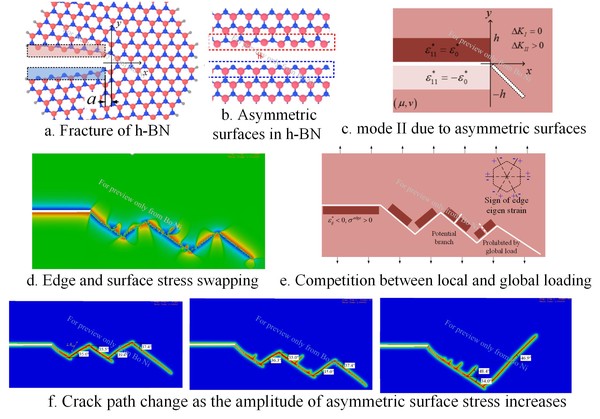
Crack propagation with lattice asymmetry in h-BN
Recent experimental measurements have discovered the fracture toughness of monolayer h-BN is much large than its intrinsic surface energy and based on DFT calculations toughening mechanisms of crack tip bifurcation and edge swapping due to the lattice asymmetry have been proposed. In this study, combining theoretical analysis and phase field (PF) modeling, we study the effect of the polarized edge stress and anisotropic fracture energy on the fracture of h-BN. It is found that polarized edge stress can contribute a mode II stress intensity factor (SIF) travelling with the crack tip and the competition between local lattice asymmetry and gloabl extrenal loading can result in a zigzag crack path with swaping branches.
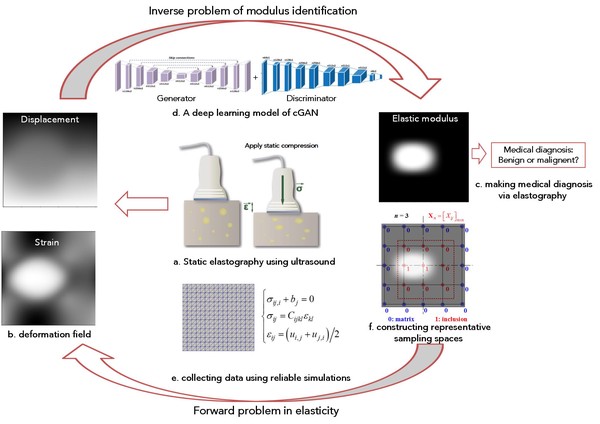
Deep learning approch to inverse problems in elastograhy
Non-destructive evaluation (NDE) of elastic modulus in materials has broad applications in fields such as geological exploration, quality evaluation and medical diagnosis. A key to these techniques is to solve the inverse problem of identifying the distribution of elastic modulus. While conventional theories and numerical methods often involve solving multiple variational problems iteratively for each individual case, the demand of real-time response and high-throughput application of NDE is growing, especially for advanced manufacturing and clinical practices. To address this challenge, in this project, we leverage some of the recent progress in data science and propose a deep learning (DL) approach to solve the inverse problem of modulus identification in elasticity for high accuracy and efficiency.
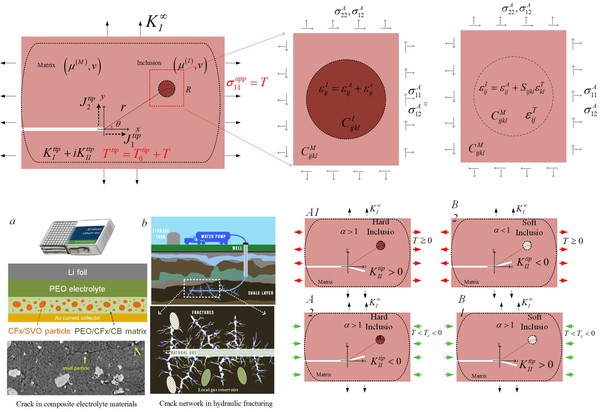
Crack-inclusion-T-stress interaction
The interaction between cracks and inclusions plays an important role in the fracture behavior of particulate composites. It is commonly recognized that an inclusion stiffer than the matrix tends to deflect an approaching crack away while a softer inclusion attracts the crack. Here, we demonstrate by analytical modeling and numerical simulations that the crack-inclusion interaction can be tuned by an applied T-stress. Under a sufficiently large compressive applied T-stress, cracks can be attracted to stiffer inclusions while repelled by softer ones, thus reversing the conventional trend. Potential applications of this work include composite electrodes in lithium-ion batteries and hydraulic fracturing.
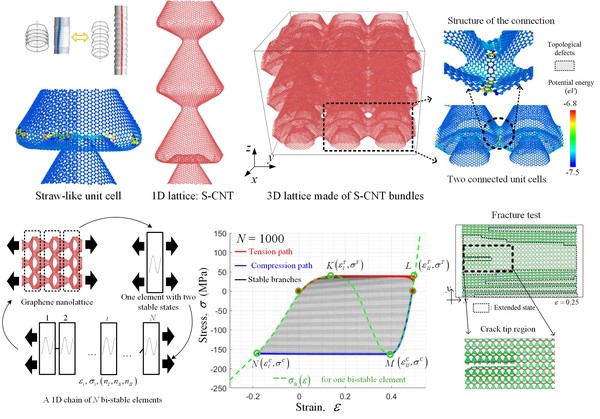
3D architectured graphene nanolattice
3D architectured graphene nanolattice has open doors to integrate superior mechanical propeties, including specififc strength, stiffness and deformability. In this project, the energy dissipation in graphene nanolattice is enhanced via a novel design with snap-through instability. We have constructed a group of reconfigurable graphene nanolattices based on a straw-like unit design. Combining molecular dynamics and theoretical model, pseudo plasticity and hysteresis of the nanolattices are demonstrated and explained. With these novel toughening mechanisms, the designed graphene nanolattice is predicted to be tolerant of crack-like flaw and dissipate energy better than carbon steel.
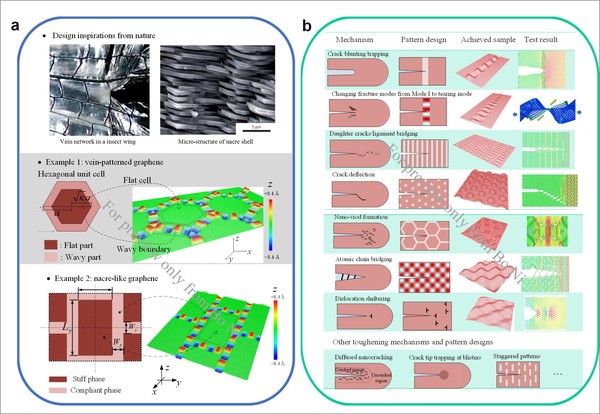
Topological toughening of graphene
Fracture has been a grave concern for practical applications of graphene other atomically thin and brittle crystalline materials. In this NSF awarded project, we have been systematically exploring the potential of using topological effects to enhance the fracture toughness of graphene. By designing topological defect distribution and controlling the out-of-plane curvature, we numerically demonstrate that various toughening mechanisms can be activated, including crack tip blunting, crack trapping, ligament bridging, crack deflection, daughter crack initiation and coalescence, pseudo-plastic deformation as well as snap-through among multi-stable states, and toughen graphene and related 2D materials effectively.